Elements of a square root and calculation step by step

Example

For example, let’s try it first with a number that is a perfect square:

Let's calculate the root of $582.169$ that is $763$ because $763\cdot 763=582.169$.

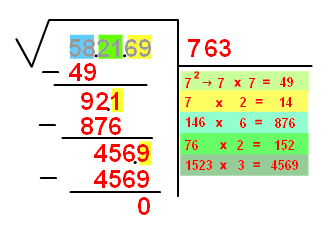
Step by step it would be:

1) We group in $2$ at a time the digits of the number $582169$, that is: $582169$. We will write it this way:

2) We look for a number that risen up to $2$ is close to $58$, in this case the number is $7$ since $7\cdot 7=49$. We will write it:

3) This result is reduced from $58$ and therefore it is: $58-49=9$ and we move down the two following digits, $21$. And we separate the last number which is $1$. We write it in this way:

4) We write the double of $7$ (that is to say $14$) in the third auxiliary line, and then the number that is on the left of the separated digit (that is $1$) is divided by $14$.

In this case $92:14$ and we obtain the closest integer which is $6$. (We write $6$ next to $7$ on the first line).

We make the subtraction, move down the next two digits and separate the last one (now $9$). It will be written as follows:

5) We do the same as in the previous step. Namely, we multiply $76$ by two (doubling it) and, $456$ is divided by this double (that is, $152$) which gives $3$.

When we do the subtraction it comes to zero and, therefore, it is not necessary to continue.

We write $3$ in the first line, and the root we were looking for is: $763$.

The square root of $582.169$ is $763$.

Example

The root of $5836,369$

1) the number of the radicand is divided in groups of two digits. The separation is done from the decimal sign (if there is one) from right to left.

If on the side of the decimals there is not an even number of digits, it is clear that a digit will be alone: in such a case, we add a zero.

If in the integer’s side we had left a number alone, it would remain like that. In our case we must add a zero next to $9$. Written, this would be:

2) We look for a number that, multiplied by itself, is $58$ or the closest minor number, bearing in mind that the result cannot be bigger than $58$.

In this case the number would be $7$, because $7\cdot 7=49$. We will write:

3) We subtract $49$ from the first group of two numbers of the root, which is $9$. We move down the following $2$ digits, that is $36$. Now we separate the last number, $6$. We will write:

4) We write the double of $7$ (that is to say $14$) in the third auxiliary line, and the number that is on the left side of the separated number (that was $6$) is divided by $14$. In this case the closest integer is $6$. (We write $6$ next to $7$ on the first line).

We subtract, move down the next two digits and separate the last one. In this case we move down $36$.

Since these are on the right side of the decimal point, in the first line (where we are writing) we put a decimal point as well. We will write:

5) Now, we repeat the previous step. We multiply $76$ by two (we double it) and $603$ is divided by this double (that is $152$) which gives $3,9$; therefore, taking the entire part, we have $3$.

The operation to be done is $1523\cdot 3$. We write the result under the last rest that we had and do the subtraction.

We move down the following two digits. We write 3 in the first line. This is written as:

6) The same previous step is repeated. We multiply the root that we have written by now $(76,3)$ by $2$ but ignoring the decimal point, that is to say $763\cdot 2=1526$.

The result is written on the following auxiliary line, and the first four numbers of the remainder $(1467)$ are divided by the result of the multiplication, giving the number $9$ as the first number that is not a zero of the division.

We write $9$ in the line of the root and $9$ is multiplied by $15269$, that is $137421$. This is the number that we have to subtract from the last remainder that was $146790$ which is $9369$.

At this point, we can say that approximately the square root of $5836,369$ is $76,39$ with a reminder of $9369$.