Equilateral hyperbola

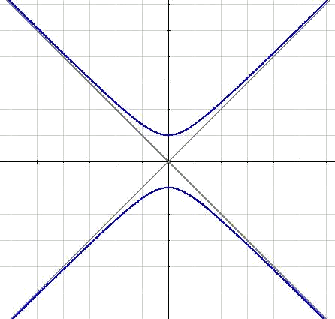
imagen

This hyperbola, in which $a = b$ , is called equilateral. Hence the eccentricity is $e = \sqrt{2}$ .

Multiplying by $a^{2}$ in the expression $\frac{x^{2}}{a^{2}} - \frac{y^{2}}{b^{2}} = 1$ , we get the equation $x^{2} - y^{2} = a^{2}$ . In this case the asymptotes would be $y = x$ , $y = - x$ .

It is possible to observe that the asymptotes are orthonormals. It would then be interesting if they were to coincide with our orthonormal axes. To do so, all we need is a $45$ degree turn. The resultant equation $x \cdot y = \frac{a^{2}}{2}$ can be expressed as $y = \frac{k}{x}$ by generating the following diagram:

imagen

Another expression, in which the hyperbola will not be in the first quadrant anymore is $y = - \frac{k}{x}$ , giving place to:

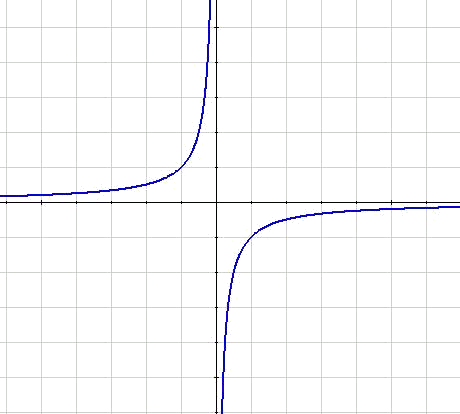
imagen

Example

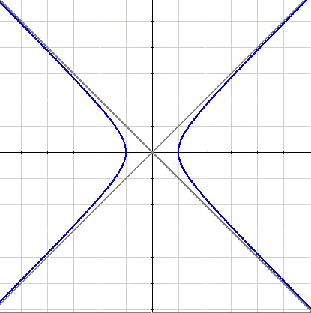
Consider the hyperbola $y = - \frac{8}{x}$ , and find its eccentricity and its focal distance.

The eccentricity is, by definition, of an equilateral hyperbola $e = \sqrt{2}$ .

To identify $k = 8 = \frac{a^{2}}{2}$ , then $a = \sqrt{16} = 4$ .

As $a = b$ , with $c^{2} = a^{2} + b^{2}$ is it $c = \sqrt{2 \cdot a^{2}} = a \sqrt{2} = 4 \sqrt{2}$ .