The function that assigns to the independent variable
We observe that if we apply the log to the exponential function base
Graph
As in case of the exponential functions, the graph of the logarithmic functions changes if the base is greater to or smaller than
Let's see it with
It is remarkable that the logarithmic functions always go thorugh the point
Example
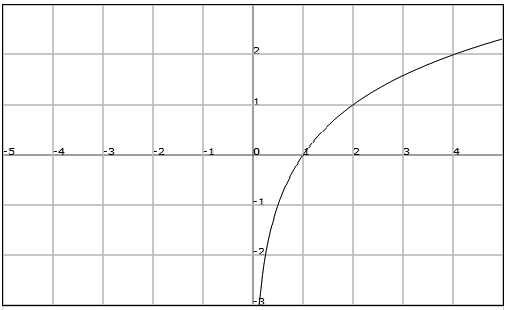
Example

Properties
From its graphic representation we observe that the logarithmic function satisfies the following properties:
- Domain:
- Image:
- Bounds: It is not bounded.
- Intersection with the axes: It cuts the horizontal axis at
- Continuity: It is continuous in its domain.
- Asimptotes: The straight line
- If
- If
- If
- Regularity: It is not periodic.
- Symmetries: It is not symmetric.
- Monotonicity: If
- Relative extrema: It does not have any.
- Injectivity and exhaustivity: It is injective (the images of different points are different), and also it is exhaustive since the image is
