The perimeter of the circle is:
The area of the circle is:
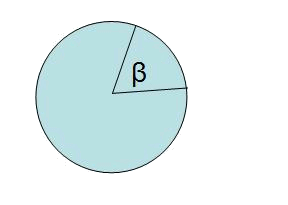
Using proportions it is possible to find the area of a circular sector and the length of the arc:
The same proportion can be used with


The perimeter of the circle is:
The area of the circle is:
Using proportions it is possible to find the area of a circular sector and the length of the arc:
The same proportion can be used with