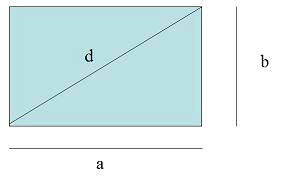
The area of the rectangle is:
Its perimeter is:
And, applying the Pythagorean theorem, its diagonal is:
Example
Calculate the diagonal and the area of a rectangle of
-
Diagonal
- Area
And so, the area of the rectangle is:


The area of the rectangle is:
Its perimeter is:
And, applying the Pythagorean theorem, its diagonal is:
Example
Calculate the diagonal and the area of a rectangle of
Diagonal
And so, the area of the rectangle is: