The rhombus

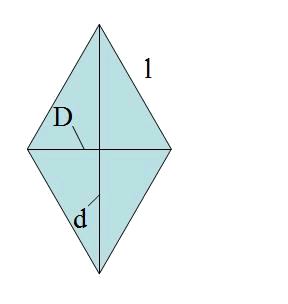
imagen

The area of the rhombus is: $A = \frac{D \cdot d}{2}$

Its perimeter is: $P = 4 \cdot l$

Example

Calculate the area of a rhombus with $D = 1 m$ and $l = 0, 7 m$

We find $d$ using the Pythagorean theorem using $D$ and $l$ :

$l^{2} = (\frac{d}{2})^{2} + (\frac{D}{2})^{2} (\frac{d}{2})^{2} = l^{2} - (\frac{D}{2})^{2} (\frac{d}{2})^{2} = 0, 7^{2} m^{2} - 0, 5^{2} m^{2} = 0, 24 m^{2} d = 2 \sqrt{0, 24} m = 0, 98 m$

The area is: $A = \frac{1 m \cdot 0^{'} 98 m}{2} = 0, 49 m^{2}$

And so, the area of the rhombus is: $0, 49 m^{2}$ .