A function defined by parts is a function whose analytic expression is not unique but rather it depends on the value of the independent variable.
Example
The function
To find the image of an element
Example
In the previous case for example,
- if
- if
- if
- if we
Since each of the expressions of the parts are defined in some domain, the domain of the function
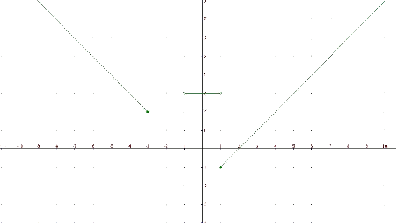
Let's see some examples of functions defined by parts:
Example
Consider the function
Its graph is the union of the graphs of the functions
The graphic representation would be:
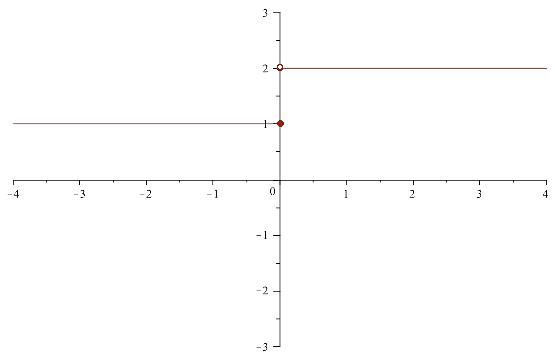
Example
Consider the function
This time its graph will be the union of a straight line, a parable and another straight line, with each one defined where it is indicated in the definition of the function.

Example
and if we want to evaluate at
Example
and if we want to evaluate at
Example
The following example is not a function by parts since the sets of the definition are not disjoint:
since for points in
